Free CBEST Math Practice Test with Questions and Answers 2026
Welcome to our free CBEST Math practice test! We're excited to help you prepare for the actual CBEST assessment. Inside, you'll find questions that are an accurate representation of what you'll see on test day.
Our Math CBEST sample questions have been carefully selected to help you improve your score, so you'll be ready to tackle the real exam with confidence. Take your time and enjoy the test, and don't hesitate to reach out if you have any questions.
Good luck!
Get more practice with our Free CBEST Practice Test or get full access to our CBEST PrepPack.
If you are looking for a different test, or are not sure which test is relevant for your position, please contact us, and we will do our best to ensure you get the most accurate preparation for your upcoming assessment.
1. 6/3 | 5/4 | 4/5 | 3/6 | 2/7 | ?
A. 1/2.
B. 1/3.
C. 3/9.
D. 1/8.
Answer:
In this series, the numerators and denominators have separate rule. Each numerator decreases by -1 and each denominator increases by +1. This means that -1 should be subtracted from the numerator (2) in the last term in the series (2/7) and +1 should be added to the denominator (7). The result is 1/8.
The answer is 1/8.
The Math section of the CBEST test includes a total of 50 questions.
2. A store is having a 20% sale on all shirts and a 10% sale on all pants. Each customer can further reduce the price of his purchase by either paying with the store’s credit card (which gives an additional 5% discount) or using coupons, but not both. If Rachel buys 2 shirts and 3 pants, and the pre-sale price of each shirt was $19 and the pre-sale price of each pair of pants was $24, what percentage coupon for shirts would be necessary in order for Rachel to pay the same amount as if she used no coupons to take advantage of the store’s credit card savings?
A. 10.5%.
B. 15.66%.
C. 22.84%.
D. 30%.
E. 35%.
Answer:
The correct answer is 15.66%.
If the pre-sale price of shirts was $19 each, then the post-sale price was 19 x 0.8 = $15.20. If the pre-sale price of pants was $24 each, then the post-sale price was 24 x 0.9 = $21.60. If Rachel buys 2 shirts and 3 pants, the price for that purchase is 2 x 15.20 + 3 x 21.60 = 30.40 + 64.80 = $95.20.
By using the store’s credit card and not using any coupons, Rachel receives a 5% discount on the total price which reduces the price to 95.20 x 0.95 = $90.44.
You are asked to find out the percentage coupon for shirts Rachel needs to use in order to pay the same amount as if she received the 5% credit card reduction.
The price Rachel would have to pay using the coupon is: (1 - C) x 30.40 + 64.80, where C represents the coupon savings.
This price should be the same as the price she would pay using the store’s credit card ($90.44), thus:
(1 - C) x 30.40 + 64.80 = 90.44
(1 - C) x 30.40 = 25.64
1 - C = 0.8434
C = 15.66%
Therefore, a 15.66% off coupon for shirts would be necessary in order for Rachel to pay the same amount as if she used the store’s credit card.
3. The following diagram describes the percentage of University applicants accepted out of all the applicants between the years 2000-2007:
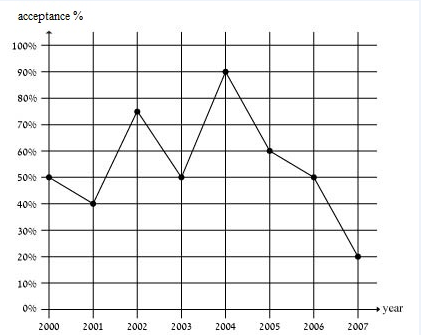
Which time period shows the biggest increase in acceptance rates?
Answer:
To answer this question, we need to find the time period showing the sharpest ascending slope.
By looking at the graph we can see that there are only two time period with an ascending slope - 2001-2002 and 2003-2004, as the last one showing an 40% increase vs a 35% increase shown between the years 2001-2002.
Thus, the correct answer is B.
The registration fee is 30$ and candidates should sign up separately to each test section.
4. 46 | 50 | 42 | 58 | 26 | ?
A. 90.
B. 84.
C. 72.
D. 60.
Answer:
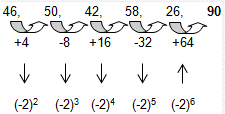
The key to solving this series lies within the intervals.
After understanding that the intervals represent a series of (-2) to the power of a number that increase by 1 in each step, we can deduce that the missing number equals:
26 + (-2)6 = 26 + 64 = 90.
Another way to look at this series would be exploring the intervals; Each time the previous interval multiplies by -2. The intervals begin at +4. Thus the intervals are:
+4
4*(-2) = -8
(-8)*(-2) = +16
16*(-2) = -32
(-32)*(-2) = +64.
Hence: 26 +64 = 90.
Estimation, Measurement, and Statistical Principles questions make up the first part of the CBEST math test.
5. A mobile company offers insurance that covers cases of theft and accidental water damage. According to their policy, the insurance pays 60% and 50%, respectively, and a $30 deductible. This means the client pays the first $30, after which the insurance pays 60% in the case of theft and 50% in the case of accidental water damage. How much will a client pay if her cell phone worth $1,080 was accidentally dropped into a glass of water?
A. 475%.
B. 500$.
C. 525$.
D. 555$.
E. 630$.
Answer:
The correct answer is (D) - $555.
The client will pay the first $30, which leaves another $1,050, of which her insurance covers 50%.
50% of $1,050 = (50/100) x 1,050 = (1/2) x 1,050 = $525
Thus, the total amount the client will pay is: $30 + $525 = $555.
Please note that you can use the “10%” method in this question:
10% of $1050 = $105
50% = 5 x 10% = 5 x 105 = $525
525 + 30 = $555
The second part of the Math test consists of computation and problem-solving questions.
6. Daily Average Number of Swimmers - Aldeburgh Beach
What was the year in which the daily average number of visiting men was the smallest?
A. 2003.
B. 2004.
C. 2005.
D. 2006.
E. Cannot say.
Answer:
To answer this question, we need to find the year with the shortest grey column, representing the number of men visitors. By looking at the graph we see that the smallest grey column is shown for the year 2003, in which only an average of 20 men a day visited the beach. Thus, the answer is A.
Food is not permitted in the testing center room, only water.
7. 0.2 | 0.5 | 2 | 2.3 | 9.2 | ? | ?
A. 9.5, 11.
B. 18.8, 25.5.
C. 9.5, 38.
D. 22.8, 24.6.
Answer:
The numbers increase by +0.3 or by x4, alternately.
The correct answer is C.
Numerical and graphing relationship questions make up the third part of the math test.
Tom completes a given task in 20 days whilst Joan requires only 12 days in order to complete the same task. Tom and Joan set out to complete one task together. They started to work at the same time; however, due to personal reasons Joan had to take a leave of absence of 4 days during their mutual work. If Tom continued working on his own during Joan's leave, how many days did it take them to complete the task?
A. 6 days.
B. 8 days.
C. 10 days.
D. 12 days.
E. 15 days.
Answer:
The correct answer is 10 days.
To solve this problem, use the following basic formula: Work = rate*time. To start, use the information obtained from the question:
Tom's work rate: 1/20
Joan's work rate: 1/12
Indicate the number of days Tom and Joan actually worked together as "y."
Begin with the four days Tom worked on his own due to Joan's leave of absence :
Work = (1/20)*4
=> Work = 1/5 => 1/5 of the work was completed by Tom alone.
This means Tom and Joan worked together to complete the remaining 4/5 of the task:
4/5 = (1/20 + 1/12)*y
4/5 = ((3 + 5)/60)*y //60 is the common denominator of 20 and 12
4/5 = (8/60)*y //Simplify (8/60) by 4
=> 4/5 = (2/15)*y //divide both sides of the equation by (2/15)
Dividing fraction by fraction is the same as multiplying a fraction by the inverse fraction:
(4/5)/(2/15) = ((2/15)/(2/15))*y =>
(4/5)*(15/2) = ((2/15)*(15/2))*y =>
(4*15)/(5*2) = ((2*15)/(15*2))*y =>
60/10 = (30/30)*y =>
=> y = 6 days
Therefore, the total amount of days it took Tom and Joan to complete the task: 4 + 6 = 10 days.
In work rate problems, unless otherwise stated, you must assume that the rate remains the same whether the two are working together or alone.
The Mathematics section of the CBEST test is comprised of multiple-choice questions only.
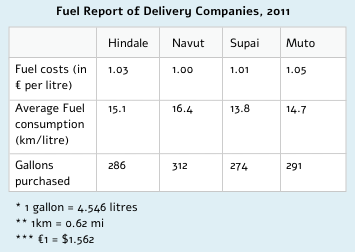
9. Approximately how many miles were covered by Muto’s drivers in 2011?
A. 9,452.
B. 11,353.
C. 12,057.
D. 15,752.
E. 19,446.
Answer:
We know the amount of gas purchased by Muto, as well as the average fuel consumption. In order to find the covered distance, we need to multiply the average consumption by the amount of gas used. The only thing we have to pay attention to is the units conversions:
(a) Muto purchased 291 gallons of fuel. Convert to litres:
291*4.546 = 1,322.89 litres.
(b) Muto averaged 14.7 km per litre. For 1,322.89 liters the distance was:
14.7*1322.89 = 19,446.48 kilometres.
(c) Convert to miles:
19446.48*0.62 = 12,056.82
CBEST Practice Test results cannot be used to predict how you might score on the actual CBEST due to differences in format and difficulty.
10. Amy and Ashley bike along the same trail. Amy bikes the entire trail at a speed of 20 mph and Ashley bikes it at a speed of 15 mph. If Amy finishes biking the entire trail 8 minutes before Ashley, how many miles long is the bike trail?
A. 0.4.
B. 2.
C. 5.
D. 8.
E. 24.
Answer:
The correct answer is 8.
According to the question, Amy’s speed is 20 mph and Ashley’s speed is 15 mph.
If Amy finishes the trail 8 minutes before Ashley, and we let t represent the amount of time it takes Amy to finish the trail, then Ashley will take t + 8 minutes to finish it.
However, since the speeds are all in miles per hour, we must convert the 8 minutes into 8/60 = 2/15 hours.
Therefore, Ashley’s actual time is (t + 2/15).
Since the distance they both bike is the same, we can now formulate the following equation comparing their times:
20t = 15(t + 2/15)
20t = 15t + 2
5t = 2
t = 0.4
We are asked to find the trail’s length, i.e. the distance they both passed. Plugging the value for t back into the distance Amy has passed, we arrive at: 20 * 0.4 = 8 miles.
You can take the test in its online proctored version or as a computer-based test in one of Pearson Vue's testing centers.
11. A loaded die is designed to roll a ‘six’ 5% more often than a normal die. The odds of rolling any of the other five numbers are even. What is the probability that the loaded die will roll at least 2 ‘six’s in 5 rolls?
A. 8.16%.
B. 21.25%.
C. 29.72%.
D. 44.28%.
E. 53.67%.
Answer:
The correct answer is 21.25%.
The simplest way to solve this question is to find the complementary event of the event described in the question, i.e. find the probability that the die will roll less than 2 ‘six’s in 5 rolls. Then we can find the probability that the die will roll at least 2 ‘six’s in 5 rolls using the following equation:
P(at least two 6’s in 5 rolls) = 1 - P(zero 6’s in 5 rolls) - P(exactly one 6 in 5 rolls).
The probability of getting a ‘six’ in a normal die is 1⁄6.
The probability of getting a ‘six’ in the loaded die is 1⁄6 *1.05 = 0.175.
Therefore, the probability of getting a number other than ‘six’ in the loaded die is 1 - 0.175 = 0.825.
The probability of not getting a ‘six’ in any of the 5 rolls equals the probability of getting a number other than ‘six’ in each of these rolls:
P(zero 6’s in 5 rolls) = 0.825 * 0.825 * 0.825 * 0.825 * 0.825 = 0.8255 ≈ 0.3822
The probability of getting exactly one ‘six’ in 5 rolls equals the probability of getting a ‘six’ in either one of the rolls and a number other than ‘six’ in all other rolls.
The probability of getting a ‘six’ in either one of the rolls equals 0.175*5. The reason for multiplying the probability of getting a ‘six’ by 5 (the number of rolls) is that we do not care in which of the 5 rolls we would get a ‘six’.
The probability of getting a number other than ‘six’ in all other rolls is 0.825 * 0.825 * 0.825 * 0.825.
Therefore, P(exactly one 6 in 5 rolls) = 0.175 * 5 * 0.8254 ≈ 0.4053
Thus, P(at least two 6’s in 5 rolls) = 1 - 0.3822 - 0.4053 = 0.2125 = 21.25%.
A scaled score of 20 to 80 is used to report the CBEST math results
12. It is well known that the CPI changes frequently throughout any calendar year. The head of the Welsh Central Statistics Bureau was eager to find patterns of consumerism. In which of the following months did he find the largest percentage increase/decrease in CPI in his country?
A. August.
B. December.
C. November.
D. May.
E. September.
Answer:
The correct answer is A.
The main challenge in this question is to distinguish between absolute and relative differences.
Although both August and September show the same absolute numerical increase, July's index is lower than that of August; therefore, the relative value of the increase in August is higher.1 out of 103.5 is a bigger portion than 1 out of 104.5, which means it is counted is a bigger percentage.
Similarly, August and December show the same absolute numerical change and more over – this change is between similar values, yet in opposite direction. As written above, an increasefrom 103.5 to 104.5 represents a higher relative change than a decrease from 104.5 to 103.5.August's value, therefore, represents a higher increase/decrease in percentages. This explains distractors B and E.
Since The head of the Welsh Central Statistics Bureau was interested in statistics in his country (as indicated in the question), only the information concerning Wales is in our interest. Failing to notice this might result in choosing distracter D as the answer, since this is the month of the greatest increase overall in the graph (England average expenses – from 100 to 105).
Solving Tips –
Usually when the solution comes in the form of a distinct unit (as months) and is not a result of some calculation, a quick glance at the distractors might save us some time. Since only five months are optional as answers, one can address only these months without examining other months as options. In our case, the greatest increase is actually in February, yet the question has just asked about "the following months".
One of the properties of relevant-selection sort of questions is the excess amount of information. It is recommended to try and focus solely on relevant information. In our case, in order to get to the solution, it is enough to examine the table's row concerning Wales. All other rows and even the graph itself is not necessary for the solution.
The passing score on the CBEST Math Section is a scaled score of 41.
13. Catharine drove 250 miles to Hertfordshire in order to spend New Year's Eve with her family. She drove at an average speed 12 miles per hour faster than her usual average speed. If she completed the trip in 1 hour less than usual, what is her usual driving speed, in miles per hour?
A. 49.1.
B. 52.4.
C. 55.
D. 61.1.
E. 65.5.
Answer:
The correct answer is 49.1 mph.
There are two ways to approach this type of question:
1. Organize the given information in a table.
When there is more than one variable we recommend representing them with reference to the one we are interested in (in this case, represent time using the velocity variable).
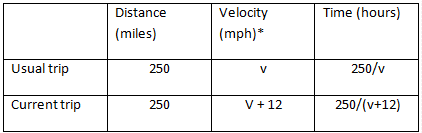
Since we are told that the current trip is 1 hour less than the usual trip we can easily form an equation using the information we have organized:
250/(v+12) = 250/v – 1 / Multiply by (v+12) and also by v
=>250v = 250*(v+12) – v*(v+12) / Open brackets
=> 250v = 250v + 3000 – v^2 - 12v
=> V^2 + 12v – 3000 = 0
In order to find the value of "v" we need to use the "Quadratic formula":
=> V1,2 = (-12 +- √[12^2 - 4*1*{-3000}])/(2*1)
=> V1,2 = (-12 +- √12144)/2 = (12 +- 110.2)/2 = 49.1 Or -61.1
Catherine could not have been driving in a negative velocity and therefore:
V = 49.1 mph
2. You can solve this problem using the basic formula: x = v*t and the given information in order to form a system of two equations-
Usual trip:
(i) 250 = v*t
Current trip:
(ii) 250 = (v+12)*(t-1)
We are interested in Catherine's velocity and therefore we will isolate "t" from the first equation:
(i) t = 250/v
And insert it into the second equation (the purpose is to remain with the velocity variable only):
(ii) 250 = (v+12)*(250/v -1)
The rest of the calculation is as shown in the first method.
*Please note that you can check yourself by inserting the velocity into the original equations and see if there is indeed a 1 hour difference between the usual and current trips. We recommend checking yourself only if you can spare the extra time.
To pass the CBEST as a whole you need to score 37 in each of the sections.
14. Which of the numbers below is closest to the correct answer?
6391 : 9.4 ~
A. 540.
B. 320.
C. 1060.
D. 720.
Answer:
6391 can be rounded up to 6400 and 9.4 can be rounded down to 9. We know that 64 / 9 ~ 7. This means that 6400 / 9 ~ 700. 720 is the closest number to the correct answer.
The correct answer is 720.
You cannot bring a cellphone or any other electronic communication devices into the testing center.
15. Imagine a square with a side length of 3 inches. If the square is divided into 9 smaller squares of 1 inch side length, what is the ratio of the perimeter of the original square to the total perimeter of the 9 smaller squares?
A. 1:9.
B. 1:3.
C. 1:1.
D. 3:1.
E. 9:1.
Answer:
The correct answer is B.
The perimeter of a square equals 4 times the length of its side. Thus, the perimeter of the original square is 3 x 4 = 12. The perimeter of one of the smaller squares is 1 x 4 = 4. There are 9 such squares, so the total perimeter of all 9 squares is 9 x 4 = 36. The ratio is 12:36 which is 1:3.
The time limit for this section is 2 hours.
16. Approximately, what is the change in rent prices per ft^3 between July and August, in Pounds?
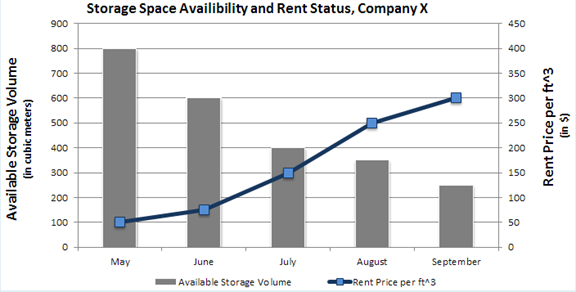
A. 32.15.
B. 64.30.
C. 75.
D. 128.60.
E. 78.
Answer:
Rent prices increased by $100 between July and August (250-150).
Using the currency exchange rate, we get 100*0.643 = £64.30
Note: Always make sure you use the correct axis:
(-) The left axis presents the available storage volume (corresponds to the grey columns).
(-) The right axis presents the rent price per ft^3 (corresponds to the blue squares).
The correct answer is B.
17. Which of the numbers below is closest to the correct answer?
34 / 1134 ~
A. 22.
B. 0.19.
C. 0.03.
D. 0.39.
Answer:
When dividing a small number by a large number the outcome is always a fraction / decimal. Therefore 22 can not be a feasible option. One way to go about this type of question is to enlarge the small number by a fraction of 10, 100 or more so that it becomes the larger number. In this case if we multiply 34 x 100 = 3400 and then divide it by 1134 the answer is roughly 3(3400/1134~ 3). We now have to divide it back by 100 (or simply add a decimal point in the correct spot ) 3 / 100 = 0.03.
The correct answer is 0.03
You can find test registration dates in the following link.
18. In rectangle ABCD, the length of the diagonal is 17 and the perimeter is 46. What is the difference between the length and the width of the rectangle?
A. 2.
B. 7.
C. 8.
D. 15.
E. 23.
Answer:
The best answer is B.
Let the length and the width be x and y. The diagonal of the rectangle divides it into two right triangles, such that x2+ y2 = 172 . The perimeter of the rectangle is 2(x + y) = 46; x + y = 23. Solve the two equations, or use the Pythagorean triplet 8,15,17; x and y are equal to 8 and 15, so the difference is 15-8 = 7.
CBEST's Mathematics section assesses your ability to perform various mathematical functions, solve problems and also your ability to utilize mathematical knowledge
| 1 | 2 | 3 | 4 | 5 | 6 |
| D | B | B | A | D | A |
| 7 | 8 | 9 | 10 | 11 | 12 |
| C | C | C | D | B | A |
| 13 | 14 | 15 | 16 | 17 | 18 |
| A | D | B | B | C | B |
Access more CBEST Practice tests and other valuable CBEST prep materials so that you take the test while being as prepared as possible:
